Mathematical Dispositions
One strategy for in-service or pre-service teachers to get into a more mathematical frame of mind and actually enjoy some interesting aspects of mathematics is to engage in games and other activities based on mathematical concepts. These are also great for beginning the school year with students. Figures 1.1 through 1.5 illustrate different types of activities including puzzles, number patterns, everyday math, and dominoes. Other activities can be found in mathematics journals, professional books, and on teacher websites.
What teacher dispositions are critical for promoting student learning of mathematics? Some are certainly global, important for all teachers: a keen personal interest in learning and growing; reflective teaching; a view that all students can learn but each student is an individual learner; high but realistic expectations of learners; flexibility in adapting instruction, materials, and assessment to varying contexts; and the ability to create a positive yet goal-oriented classroom environment. Some dispositions are more specific to mathematics content: genuine interest in mathematical concepts and connections; a persistence with finding solutions to problems; the willingness to consider multiple processes or multiple solutions to the same problem; and an appreciation for mathematics-related applications such as those in music, art, architecture, geography, demographics, or technology.
Figure 1.1 Picture Puzzles | |
1. How many 2 by 1 by 1 blocks (length by width by height) make up this structure if at least one face of each block is visible? How many 1 by 1 by 1 blocks would be required to make the same structure? 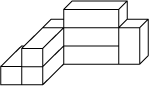 | 2. Here is a pattern for making a cube (three-dimensional shape with six squares for sides – such as a die). The thin "tabs" are to be folded for gluing. Using a piece of graph paper, design another pattern for a cube. Or try another shape, like a pyramid or tetrahedron. 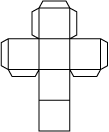 |
Figure 1.2 Fun with Fibonacci Numbers | |
Fibonacci numbers are numbers of the following sequence: 1, 1, 2, 3, 5, 8, 13, 21 ... where successive numbers are the sum of the two preceding numbers. This sequence of numbers or patterns formed by this sequence appears naturally in the environment. Find a leaf, stem, pinecone, or seashell with the spiral pattern shown here. Look for this pattern elsewhere in nature or art. Draw your own spiral on a piece of graph paper following these direction.
That spiral is a Fibonacci pattern! | 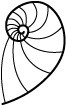 |
Figure 1.3 Other Number Patterns | |
1. Predict the number that would come next. What is each pattern?
| |
| Using a hundreds chart (1 to 100), explore the concepts of multiples and factors (prime and composite numbers):
| |
Figure 1.4 Math All around Us | |
1. Take a walk around your neighborhood and make a list of all the mathematics application you spot.
2. Look through a newspaper and make a list of all the math applications (obvious and hidden). Some examples of obvious application: weather charts, stock market, sports statistics, grocery sales. Hidden applications: probability of events, statistics within an article, graphs, reports on science and technology.  | |
Figure 1.5 Dominoes: A Common Game with Powerful Math Concepts | |
Obtain a set of 28 dominoes, or print and cut out a set. Study the dot patterns and count up the groupings. The pieces are sometimes called bones, the dots are called pips. Any group of bones with a common end is called a suit, and bones with identical ends are called doublets.  Make up a game for two players or follow the rules below for a simple game. (More complex rules can be found online or in a book on dominoes.)
| |







